Physician's Notebooks 2 - http://physiciansnotebook.blogspot.com - See Homepage
Reading the following section may increase your scores on tests - IQ, SAT, MCAT, TOEFL - and get you a free or very low cost education scholarship.
2.6e: Update 15 November 2021

Note; If you are studying to learn arithmetic, to become an idiot savant in it, read slowly in small segments and re read when you do not at first get it. And at the end of a segment, contemplate what you have just read and, briefly, try to sleep on it.

Numbers for Healthy Longevity

Contents
(Below are in order as in text. Use search & find or scroll for topic)

Numbers

Zero

Infinity

Unity

Number Systems

Binary System
Brief History of Mathematics

Electronic calculators
Microsoft Excel
Digital Electronic

Mental Marvel Day for Date

Nine-Remainders of Number, the C-S

Eleven-Remainders

Mental Math

Addition
Subtraction
Multiplication
Multiplication without multiplying
Division
Fractions
Decimals
Percents
Signed Numbers
Order of Operations and use of Brackets

Exponents, Squares, Cubes, Roots, Scientific Notation (SN), Logarithms

Prime numbers

Perfect Numbers

Statistics
Statistically Significant

Geometry

The Fourth Dimension
Tesseract

Visualizing Acres

Numbers: Start by counting 1, 2, 3,... . The “One” means wholeness or singularity. In our world “one” is the most natural of things. Just about everything we know, including ourselves exists as ones, or wholes. The number system could never have started without the concept of one. So prehistoric Homo sapiens looked at her or his fingers and started counting.

Except zero, the numbers are called, natural numbers.

If you include zero with the natural numbers, you get the set of whole numbers; each has meaning of being the pure, exact, singular number itself and not an approximation, not a mixed fraction nor a decimal. Integer is another word for whole number.

Numbers connect with wholeness because based on whole units. For example, One and a half (1 & 1/2) human may be imagined anatomically but could not exist alive. There are 1, 2, 3, etc. real persons and not mixed fractions of them. And the quantum theory states that matter and energy at its most basic level is composed of indivisible 1 units - quanta (singular quantum).

When we make measurements that count singular things – like a population census or a count of how many persons died from cancer in New York State in 1998, we should come up with a whole number, whatever it might be. On the other hand, if I ask you to measure the length of a defined distance, each repeat measurement will differ a bit due to the essential inaccuracy of your measure, and you will end up with an averaged result like 12.03, which is not a whole number but a mixed decimal number.

Zero: Only numbers 1 to 9 of the set of natural numbers are what we, strictly, call a digit, but, practically, the zero is an essential part of the digit numbering system. "Digit” relates to our fingers or toes, on which primitive humans started to count.

Our present number system is based on the ten-count from humans having ten fingers or toes. But this system was not the original one in Europe. The ten-count was first used in India and adopted by the Arabs. The old English system was based on the 12-count. This has led some to speculate that extraterrestrial visitors (ETs) with left and right 6-digit extremities introduced the system, an interesting idea that awaits proof in the form of a rusting spaceship that can be isotope-dated back 20,000+ years!

Infinity in mathematics is written like 8 on its side (∞). Infinity is not just a very, very large number. It means The End; no more numbers left to count. If we imagine an imperishable robot starting to count from 1 at the instant of the Big Bang Creation, then infinity could be imagined as the last number counted before everything ends in silent blankness forever.

Unity: If the Universe will eventually contract (the Big Crunch) back to its beginning when it was a primordial condensed unit, the idea of infinity will become unity, or 1, because at that instant, just prior to the next Big Bang, everything that exists will be part of the One.

Number Systems: With civilization, the number systems evolved. Our number system is called "the decimal system" because based on ten. When we count the first ten whole numbers: 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, we use a different sign for each. But when we write 10 we recycle a couple of already used signs. In the system, we have agreed that moving a figure one position to our left means multiply by ten. The zero in 10 tells that the digit 1 has been moved one position to left compared to its position in the number 1, and the number is ten. When we see a figure like 567, we know automatically that the 5-digit in this position means 500 and that the whole 567 is a condensed way of writing “five times a hundred plus six times ten plus seven times one” and summing to five-hundred, sixty-seven.
A number system need not be limited to ten. It only happened that way because the humans who originated it used their ten fingers for counting. In the system based on twelve, the duodecimal, we could still count 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, but the figure after 9 would not be written 10. Instead, we could place the Roman numeral for ten, X and call it “dek”. Then for eleven, instead of 11, we could use another single sign, the Greek Σ, said “el”. So our counting system based on 12 would be 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, X, Σ. In the 12-digit system, the first double-digit is the twelve-count, and could also be written 10 but spoken "twelve". From there-on, the system looks the same as the decimal except that X substitutes for 10 in the decimal system and Σ substitutes for 11. In the duodecimal system the 10 is equivalent to our 12, and the 11 to our 13 and so on.

The duodecimal system uses the 12^th whole number (written, 10) for magnitudes instead of the 10th of the decimal system. So duodecimal 10 becomes equivalent to decimal system 12, and duodecimal 20 is equivalent to decimal 24, and so on. And duodecimal “100” is decimal “120”. Thus, 567 in the duodecimal system is a number in the decimal system whose count is (5x12²) + (6x12¹) + (7x12⁰), or (5x144)+(6x12)+7x1=720+72+7, or 799.
Any set of counting numbers can be selected for a number system. For example, based on 2 digits, a 2-count binary system is popular in our computer age.
The Binary System is a series of zeros and ones. In Binary, counting from Arabic numbers 1 to 15 is 01, 10, 11, 100, 101, 110, 111, 1000, 1001, 1010, 1011, 1100, 1101, 1110, 1111.

To convert binaries to Arabic numbers use 3 rules:
1) Each binary 0 is the same value as our usual zero.
2) Each binary 1 is an Arabic 2 with an increasing exponent – 2⁰, 2¹, 2², 2³, ... 2^(n-1). In the exponent (n - 1, or n minus 1), the n is the number position of the binary number 1 in the number count starting to your right. For example, in 01, n=1 for the 1 in position to your right so for the 01 it is 0 + 2^(1-1), which computes to 1. For position 2 as in 10, it is 2^(2-1)+ 0, which is 2.
3) Each 0 or 1 in binary system should be visualized with a plus sign (+) between 0’s and 1’s, keeping in mind that a binary 1 is the Arabic 2^(n-1). More examples: binary 11 is 2¹ + 2⁰ = 3, binary 100 is 2² + 0 + 0 = 4, and so on.

As you see, the larger the Arabic number, the longer the binary series of 0's and 1's.

Binary has the advantage to be used as an alternating electronic off-on switch in a transistor or computer chip, where a zero (0) signals switch-off and a 1 signals switch-on. The binary system is what electronic digital computing depends on.

Roman Numerals are not really a number system but the Romans used them to depict numbers. And, today, we see them usually after copyright dates. They are as follows.

1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, ... , 21, ..., 101, ..., 1001
Roman I, II, III, IV, V, VI, VII, VIII, VIIII, X, XI, ..., XXI,..., CI...,MI
So typically,we would see the year as it is today MMXVIII or 2018

Brief History of Mathematics: The story goes back to when humans first started counting. Some groups used the 10 fingers, other (French culture) the 20 fingers and toes, and the English-speaking area used 12 counts (for unknown reason; 6-finger hand alien contact?) The ancient Egyptians pioneered because of the yearly planting of crops giving rise to geometry around the Nile River. From then the Ancient Greeks picked up, starting with Thales in 6th century BC. Interestingly he was a traveling salesman, internationally, and by frequently going to Egypt picked up the new knowledge. His famous pupil was Pythagoras whom most of us still know today because of his so-named theorem (See later in the chapter, his magic triangles). By 300 BC, Euclid came along and wrote a series of texts and established theorems and really set mathematics on its modern course. Finally, in this Ancient Greek group was Archimedes, who died famously by a Roman sword in Sicily at the siege of Syracuse in 212 BC.
Meanwhile, more to the east, the Hindus were inventing the place number system by discovering the use of the Zero. They passed it on to the Baghdad Arabs and from the Arabs we got the system of Arabic Number and things really started moving in calculation.

Electronic Calculators: First, give thought, Do I need one personally? Most offices are supplied with many, your cell phone has one, and every personal computer has advanced calculator function on Google.
Many computer users are not aware that the Microsoft Excel has very advanced calculator function. Go to Excel and note the fx line space toward the top left. The following symbols are used for calculator function: the usual + and - in their normal functions; and the * for the x multiplier, the / for division, the ^ after the number for its exponent, and brackets for complex functions. All you need do is type in your equation on the Excel fx line starting with the = and finishing with hitting the Enter key and you will see your number answer to 9 decimal digits if needed, in the A column, from line 1 down. For example, to multiply 2 x 3 = 6, you do =2*3, hit the Enter and you will see the answer 6 in the A column. For a division of 6 by 3, do =6/3 and hit the Enter and you see the answer 2. For complex functions with brackets, such as you need the M keys for in digital calculators, you just use the given symbols, e.g., for (2 + 3) x (3 x 4) =, on Excel you do =(2+3)*(3*4) and hit the Enter and you will see the answer 60 in the A column. For exponential calculation, you use the ^exponent symbol; for example, the number 136 multiplied to the 6th power is given by =136^6 and hit the Enter, and you will see the correct 3.28985 E+12 in the A column; or for the 5th root of 33, you do =33^(1/5) and hit the Enter and the correct answer in Column A will be shown as 2.012346617.
You may also use the Google type online. In that case, the symbols are all the same except that the = sign is typed in at the end as you would normally do in paper calculation.
The Excel allows advanced calculator function without the Internet access you need for Google.
If you must buy a digital electronic calculator, buy inexpensive. The following info on keys applies to digital calculators.
The AC (All Clear) key clears all previous calculation and the C (Clear) key clears only the last key that you have just digited. The C key is useful when you do a calculation involving more than two parts - Say 3 + 4 + 5? - and you mistakenly press a key - Say the 8 key? - instead of the correct 5 key (You do not need the + key to correct, just the digit, here the 5 key). By pressing the C key immediately after your mistake, you delete only the mistaken 8 from your calculation and can replace it with the correct 5 key and continue the calculation without having disturbed your calculation.

The following special key functions are taken from the inexpensive 10-digit Casio calculator. For special keys not mentioned here, check Google.

Memory Function Keys (M+, M-, MR, MC {MR & MC may be MRC} & MS)
MS is Memory Store; it puts the number into the memory. (It may be absent from some calculators with other M keys; the below M+ and M- do MS)

M+ is Memory Add; it takes a calculation and puts it in the memory in a plus form, e.g., Say you want (3 x 4) - 24 = ? First, key-in 3 x 4, then the M+; then key-in the quantity (MR - 24), i.e., minus 24, and the equals sign (=) will give -12 (minus 12).

Similarly, M- is Memory Subtract; it takes a calculation on the display and puts it in memory in a minus form. Say you want (minus) - (3 x 4) - 24 =? First, key-in 3 x 4, then the M-, then key-in the MR - 24 = -36. (Note you could also do this as (-3 x 4) - 24, keying in -3 x 4, then the M+, then key in the MR - 24.)

MR is Memory Recall; it recalls the calculation you put in the memory and, as shown above, is the = key for M key functions.
MC is Memory Clear; it sets the memory to 0. (Note, it is for the memory keys only; not the GT key or normal calculation)

For multiplying or dividing one number repeatedly by many numbers: for example, if you want to multiply by the constant pi (3.14159):
Key in 3.14159. Then MS (stores the number; if your calculator lacks the MS key, use the M+ key in its place to store the positive number) then do as many multiplications of the pi as you need, using the MR key for the stored pi number.
You could do the same with division, addition or subtraction or combinations.

The M buttons are meant to be handy for doing complicated bracketed calculations. For example, say you wish to calculate (4+3) + (3 x 2) – (6-4)? You key 4 + 3, then M+; 3 x 2, then M+; 6 – 4, then M-; and, lastly MR, and the answer 11. And if you wish to do a next bracketed calculation, you should press the MC after your MR result to clear the memory calculation. If you do not clear it, the last memory calculation will be added on to your next one to give a mistaken result.

The GT (Grand Total on most Casio calculators) key when pressed after the = key, stores the = calculation number in memory. To clear it, you must press AC. It may also be used in complicated bracketed calculations; for example, say you added 2 + 3 = 5; then you want to add-on another calculation like 2 x 3 = 6 [In bracketed form it is (2+3) + (2 x 3) =]. On the calculator you press 2 + 3 =5, then press GT (holds the 5 in memory) and then do 2 x 3 + GT = and you'll get the correct answer, 11. After you finish using a GT calculation, always clear the GT by pressing AC key; otherwise it may screw up your usual calculation.

The GT key is really unnecessary if you have the M keys and just adds complications if you forget to clear it after use.
Finally, the solid right-pointing arrowhead, or triangle pointing to your right side that you may note in the top row of your Casio is for removing the most rightward digit in a multi-digit number you are keying-in but incorrectly have entered the wrong digit. It removes the final digit of your just-keyed-in number and allows you to key-in the correct digit without having to clear.

The inexpensive calculators often have 2 sliding controls in the topmost row. In the Casio these are as follows: The [F CUT 5/4] and the [4 2 1 0 ADDsubscript2] (The number-digits on some Casio's may differ slightly)

What is the [F CUT 5/4] switch for? In a calculation involving decimals, this switch controls rounding off to a certain number of decimal places. The F gives floating decimal (the result of the calculation fills the maximal digit decimal places on the screen not including terminal 0's); the CUT cuts it off to 4 decimal places; and, in the 5/4, the calculation will not change if the digit after the one you are rounding to is 4 or lower, but will increase round up one digit if the terminal digit is 5 or higher. For usual operation, set it at F.
What is the [4 2 1 0 ADD (sub2)] switch used for? This switch is used to determine how many decimal places your answer will be displayed in. But it will not work if the [F CUT 5/4] switch is set on the F. The ADD 2 is often used for money calculations, so that it is not necessary to enter the decimal point. It automatically places 2 decimal places from the end for the cents of dollars and cents. For example, enter 123 and, with the slide on ADD 2, press the = key and the display will show ($)1.23. At opposite end, slide left to 4, enter 123 and you get 123.0000, to the 4 decimal places. (Again note your decimal switch should be set to CUT or 5/4). For usual operation set switch 4. (End of Electronic Calculators)

Mental Marvel, Day for Date. For example to answer "What day was I born?" almost instantly.
The system starts with the 12-digit 743 752 741 631 (I recall it with the nonsense phrase "Cream, clean, creed, Jim ate"; Jim being my son, and I convert the consonant sounds into numbers by my system) which are the calendar date 1st Sundays of each successive month of the key years of the 20th Century (1900 to 1999; the key years are 1912, 1940, 1968 & 1996); or, in the 21st C, the 1st Saturdays; or, in the 19th C, the first Tuesdays; or in 18th C, the 1st Thursdays. And note the 2012, a key year of the 21st C so you may quickly figure what weekday for any 2012 calendar date; by using the 743 752 741 631 you get each month's first Saturday date - 7 Jan, 4 Feb, 3 March, 7 April, 5 May, 2 June, 7 July, 4 Aug, 1 Sept, 6 Oct, 3 Nov and 1 Dec.

Note that as the centuries progress the 1st weekday of the months, which one got by use of the above-shown 12-digit; these, regress each successive century in the pattern Thurs - Tues - Sun - Sat. Also note the 28-year intervals in 12-40-68-96.
The System in Action: Say I ask "What day of week was I born?" and my birth date is Jan. 10 1933? First I take the closest key year - 1940 - and see its first Sunday, 7 Jan. 1940, and move up 3 days to 10 Jan. 1940, a Wednesday. Then I click (go but, like the computer, in my brain I click) back 7 years, moving in the backward direction for day of week and including an extra day when I cross a leap year. So I click back from 10 Jan. 1940, Wed., to 10 Jan. 1939, Tues., to 10 Jan. 1938, Mon., to 10 Jan 1937, Sun, to 10 Jan. 1936, Fri., (a leap year crossed), to 10 Jan. 1935, Thurs., to 10 Jan. 1934, Wed., and at last to my birth date 10 Jan. 1933, a Tuesday. (If you check by Google, it is correct). Also be aware that the 00 century years can only be leap years if each one is divisible by 4 (Year 2000 a leap year, 1900 not a leap year).
Knowing the 12-digit, you can locate any weekday in the Gregorian Calendar. (It started 15 Oct. 1582 in Catholic Europe but many countries did not adopt it till later - in UK, 1752, and lastly in Russia, 1917.) And you may go into the Julian calendar (last day was 4 Oct. 1582) by applying the correction and into the future for as long as we continue to use the Gregorian calendar and the present 7-days-of-week system.

"Of what use is this other than parlor-party trick?" you may ask. First, even so, it is an example of how your mind can work like a computer. Second, even though by Google, you may find any day of week for date, it may come in useful when you need to quickly determine a name-day in the past or future and are not at a computer.
For an entertaining seminar, click 3.(21-22) Seminar 3 - Day of Week .

Nine-Remainders of Numbers - the Cross-Sum: Did you ever think to add up the digits of a number – any number? For example, the digits in the numbers 23 add to 5, number 27 to 9, and 16 to 7. With larger numbers you get multiple-digit sums, which, if you keep adding up, give a final single-digit sum, e.g., 7,632 gives a digit-sum 7+6+3+2=18, and then 1+8=9

The final single-digit sum of a number is its cross-sum (CS). In cross-summing, the digit 9 is same as 0, as can be seen from the example of CS 11, 119, 1919 and 91199. We can ignore 9 or 9-sums in a number when obtaining the CS. So in cross-summing a number, 9 equals 0 and numbers that sum to 9 cancel out as 0.

This makes CS fast for many long numbers. For example, just a glance at the number 1,954,720 tells its CS is 1 since you may cast out the 9, the 54, and the 720 as all being equivalent to 0, leaving 1 as the CS.

“Alright”, you may be muttering. “Very nice, but what use?”

First, since the CS of a number turns out to be the single-digit remainder after you divide that number by 9, then any number with a CS of 0 (recall that 0=9 in CS) is exactly divisible by 9 (Check with your calculator if you don’t believe), and if the CS is 3, the number is exactly divisible by 3. So you now have a quick method to find numbers that can be exactly divided by 3 and 9. Useful for a school kid on arithmetic test.

But CS-use extends further. The CS of the answer of any arithmetic (addition, subtraction, multiplication, division) always equals the CS of the numbers used in it. For example, 32 + 41 = 73, if converted to CS is 5 + 5 = 10, and reduced further 10 = 10, or finally 1 = 1. Or take a multiplication 32 x 10 = 320, reduced to CS is 5 x 1 = 5, or 5 = 5.

Since this is the case always, it is a quick way to check for error, which can occur even when you use a calculator, if you hit a wrong key.

To check: You do your computation and check it in the CS. If there is an error, e,g., you 151 + 322 on your calculator but, by error, you digit the 6 instead of the 5 key and come up with an error 483 instead of the correct 473. The answer you got, 483 shows CS 6, so you know you have an error.

"If the CS numbers of a computation do not show an equality doing the computation, the answer is in error" is the basis of this test. That is always the case, making this an absolute test of error. But, does it mean that if the CS numbers on each side of a computation are equal, it is always a correct computation?
No. It does not always follow. An equality of the CS computation, most of the time, means a correct computation but, errors of magnitude (10's) are not picked up (e.g. 38 x 25 = 950 is correct and the CS test confirms it, but 38 x 25 = 9500, an error of magnitude, also shows a CS test giving a CS equality; yet obviously it is wrong). An order of magnitude check will quickly pick up the error. (Here by sight mentally, but in larger numbers by rounding off to nearest hundreds or thousands and mental multiplication; in the above example 38 x 25 may be rounded to 40 x 25 which can easily be mentally computed to 1,000 showing at once the 9,500 is incorrect) Also errors involving a difference of 9 in the CS of the answer will show CS equality yet be in error (e.g., 38 x 25 = 9950, sometimes a result of a computer glitch that duplicates digits).

For a while, to get the swing of it, start using CS as a routine. Get into the habit of automatically getting the CS of every number you see. It’s fun and will help you better to succeed at the game of life.

Eleven,-Remainders: Another interesting system is the remainder after dividing numbers by 11. To get the 11-remainder, take any number and subtract the sum of its even digits from the sum of its odd digits. In counting, odd or even starts at the unit, or last non-decimal digit of the number. In 1,234, the unit digit is the 4 (odd); the 3 is the 2^nd (even) digit, the 2 is the 3^rd; and the 1 is the 4^th; so the 11-remainder is (4+2) – (3+1), or 2, and you may do the division of 11÷1234 yourself to confirm. If the 11-remainder calculation is a negative, then, one converts it to a positive by adding 11, e.g., the 11-remainder of 230 is (0+2)-3, or –1, a negative result that is converted to the positive sign 11-remainder by doing a + 11 to give 10, which if you do the actual 11÷230, you will see is correct.

The usefulness of the 11-remainder system is that it is a quick way to find a clean multiple of 11 by getting an 11-remainder that is 0, e.g., 132 has an 11-remainder of 0, therefore it is a product of the 11 multiplication table (13x11=132). This can be useful in factoring large numbers and as a test for prime numbers. Also, the 11-remainders can be used like the 9-remainders to test for error in computation and using both remainder systems together in testing reduces the error noted above with the 9-remainder test.

Mental Math is good for your brain and useful too. Addition is helped by splitting numbers; it is best with 3 or 4 digit numbers. So instead of holding 3846 + 2456 in one's head, split to 38 46 + 24 56. (Spoken with pause between 38 and 46) Test it and see - split numbers are easier to compute mentally. When you do splits of unequal length, in your mind line up the numbers, e.g., 3846 + 252 as 38 46 + 2 52, with the 46 exactly over the 52 and the 8 of the 38 exactly over the 2.

And do not repeat in speech what you are computing mentally. When you add (1+2+3+4), get in habit of speaking only the partial and final answers and not reciting what you do mentally. Instead of reciting “one and two are three” “three plus three equals six” ..., say “three” (the sum of 1+2), “six” (sum of the 1^st partial sum +3), and “ten”.

In adding, look for digits that make a ten sum or tens or hundreds multiple (e,.g., 6 and 4, 17 and 3, 137 and 163) and then mentally assort for rapid calculation. In 13+15+17+15, your mind should do (13+17) + (15+15) for speed and accuracy.

Subtraction may be done by splitting and working from your left. For example 5827 minus 4414, changed to 58 27 minus 44 14 can at once be seen to equal 14 13.
Multiplication First the signs that mean multiplication or 'times". The small x, the dot. or the asterisk * can all be multiplication signs, and also simply apposing two numbers; thus if we take a and b as two different numbers, a x b, a.b, a*b and ab, each, mathematically, may mean the number a multiplied by the number b.
Multiplication is helped by knowing key number 10 multipliers: For 5 you just halve the number being multiplied and multiply by 10, which is simply appending 0 for the answer (22 x 5 is 11 x 10 = 110). For 25 you quarter the number it multiplies and append two zeroes (24 x 25 = 600). For 50 you halve and append 00. For 125 you eighth the number and append 000 (64 x 125 = 8000). OK, now a trick.

Multiplication by 11 keeps the first and last digit as a frame and adds the sums of each consecutive two digits. Example: 11 x 5,413 will be framed with a 5 and a 3. So the answer will be five digits in the order 5 and (5+4) and (4+1) and (1+3) and 3, or 59,543. With 11 x 6,413, the answer is five digits in the order 6 and (6+4) and (4+1) and (1+3), but since the 6+4 is 10, you carry over the 1; so the answer is 70,543.

Multiplying by 9 is done appending a 0 to the number being multiplied then subtracting the number from it. Example: 9 x 3,456 is 34,560 minus 3,456, or 31,104.

Multiplying by 15, halve the multiplied number, add it to the number and multiply by 10 by appending 0. Example: 15 x 38 is 19+38, or 57, and append 0 for 570.

Sliding Method for Mental Multiplication: Most useful for doing 2-digit number multiplication. In the below example, the multiplication is 48 x 76. In the method, one multiplier is reversed (Here 48 is reversed to 84), positioned so that the smallest unit digit of the reversed number (here, 4) is out on the right-side end of the multiplication, and then the reversed number slid (here, toward your left over the 76). Then do the multiplication starting at the a column where the 8 of the 84 tops the 6 of the 76, then click slide as under b where 84 is flush over 76, and finally to the c column. (This example for 2-digit number multiplication)

　a b c

84 84 84 Units: 8 x 6 48

76 76 76 T(4x6) + (8x7) 80

Hund: 4 x 7 28---

48 x 76 = 3648

Multiplication without Multiplying: Here is a curious, useful way of multiplying by only halving, doubling, and adding. It was used by illiterate Russian peasants to calculate cash value of crops: The two numbers to be multiplied are placed side by side, smaller number on left and it is halved until 1. (When odd numbers are halved here, the next lowest integer is listed) The number on right gets doubled as many times as the number on the left is halved.Then in the right column, add only the numbers next to the odd numbers in the left column. The result will be the correct product of the two original numbers. The method for 259 X 376 is below. Note that the 259, the 129 and the 1 are the odd numbers of left column and that adding up the three numbers across from each of these in the right column gives the correct answer.

259 multiplied by 376 = 97,384

259 by + 376*

129 +752*

64 out 1504

32 out 3008

16 out 6016

8 out 12032

4 out 24064

2 out 48128

1 +96256*

97384
(Note the * points the right column numbers to be added)

Division; Includes Fractions and Decimals

Division symbols & terms In ‘6 divided by 2 equals 3', division can be expressed as 6÷2, or 6/2. The words are: six (the dividend, the amount you want to divide) ÷ (divided by) two (the divisor) =(equals) three (the quotient).

Division reverses multiplication, e.g. 2 x 3 = 6 reverses to 6÷2=3. When you look at a division, e.g. 6÷2, see the factors of 6, (2)(3).

Rules for Divisibility: A number is divisible

By 2: All even numbers.

By 4 & 8: No odd numbers. By 4 if its last two digits form a number divisible by 4 or 8, and by 8, if last 3 digits are divisible by 8.

By 3 – 6 – 9, if the 1^st cross-sum of its digits are divisible by 3 – 6 – 9: thus, 456 (4+5+6 =15), divisible by 3; 426, by 6 & 3; and 81135, by 9 & 3.
By 5 if the last digit is 0 or 5.
By 9 if the cross sum of the number is 9

By 10 if number ends in 0.

By 11 if its 11-remainder is zero.

By 15, if divisible by 3 and by 5; by 24, if divisible by 3 and 8.

By 25 if its last two digits are 25, 50, 75, or 00

Special cases. 7, 11 & 13: factors of the fabulous 1001. A trick in divisibility for 7, 11 and 13 divisors is based on their being the prime factors of the product 1001 (7 x 11 x 13=1001). And if you multiply any 1-digit or 2-digit number by 1001, you get a mirror-image number (1001 x 5=5005, 1001 x 53=53053 and every product of 1001 multiplication is divisible by 7, 11 and 13. So up to the highest 5-digit number (99999) you may quickly find out if it is divisible by 7, 11 or 13, by simply subtracting its next lowest mirror image (eg, 99999-99099=900) and testing the remainder for divisibility by 7, 11 or 13.

Fractions: In writing a fraction, I use diagonal slash, equivalent to the usual mid line horizontal bar: on the left is a numerator, on right, a denominator. The numerator can be any whole number but the denominator cannot be 0. A fraction represents division of numerator by denominator. Thus in 1/2, a 1-unit is being divided into 2 equal parts. You can visualize a cake divided into two semicircles.

The equality a/a = 1 is important: it says that same terms in a numerator and denominator cancel out. Number-wise, in division the example 144/108, partially factored becomes (12)(12)/(12)(9), and since 12/12 is a form of a/a=1, the term cancels out since (1)(12/9) is the same as 12/9. Similarly, 12/9 can be factored to (3)(4)/(3)(3) and a canceling out of like terms give the final 4/3.

Fractions like 4/3, made of a whole number and a fraction, e.g., 4/3 is 3/3 + 1/3, or 1 + 1/3, which we can write 1 1/3, are called “improper fraction” because they are made of a whole number and a fraction, e.g., 4/3 is 3/3 + 1/3, or 1 + 1/3, which we can write 1 1/3. A whole number mixed with a fraction is called “mixed number.”

To change improper fraction to mixed number, divide numerator by denominator and then express the mixed number as the whole-number of the answer (quotient) followed by a fraction of the remainder over divisor. Example, improper fraction 3/2 is 3÷2, giving whole number 1 with remainder 1, so mixed number is 1 1/2.

To add, subtract, multiply or divide using mixed numbers, convert them to fractions.

Fraction Multiplication: To multiply two fractions, multiply numerators and denominators as follows: (a/b)(c/d) = (a•c)/(b•d), or substituting numbers 2/5 x 2/7 = (2 x 2)/(5 x 7)=4/35.

Equal fractions may be expressed using various numbers, e.g., 1/3 = 2/6 = 3/9. Then it is useful to find Lowest Common Denominator (LCD). For example, in 1/3, 1/6 and 1/12, the LCD is 3 but what about in 1/3 and 1/5? The LCD is not 3 here because it does not divide into 5. First inspect 1/3 and 1/5 to see if you can spot an LCD. If not, bring the fractions to higher number denominator by multiplying the two denominators [3 X 5; e.g., (1/3)(5/5)= 5/15, and 1/5(3/3)= 3/15] and get the LCD, here 15. Thus, when not obvious, you find an LCD by multiplying both denominators. What about 7/12 and 4/15? Here look at each denominator to see if you can factor to primes. In the case the just given, 12 =(2)(2)(3) and 15=(3)(5); and the number 2 appears twice as a prime factor, so it may be expressed as 2². Thus we have the prime factors expressed as 2², 3 and 5. Multiply all the prime factors here together, 2 x 2 x 3 x 5=60, and you have the LCD of 60.

In subtraction or addition, the fractions involved must always be in the form of an LCD. Once you have fractions in an LCD, addition and subtraction are easy, e.g., a/LCD + b/LCD = (a+b)/LCD; and a/LCD minus b/LCD = (a – b)/LCD. Taking the above number fractions as example: 7/12 (7/2²x 3) + 4/15, 4/(3 x 5) = 35/60 + 16/60 = 51/60.

Division by Fractions: To divide by a fraction, invert the dividing fraction and multiply as follows:

a/b ÷ c/d = a/b•d/c = ad/bc; or 1/2 ÷ 1/4 = 1/2 •4/1, or 1/2 x 4 = (4 x 1)/2 = 2.

Decimal comes from, ‘decimal fraction’, whose denominator is power of 10, e.g., 3/10¹ = 3/10 = 0.3 (Read as “3-tenths” or “zero point three”); 3/10² = 3/100 = 0.03 (Read “3-hundreds” or “zero point zero three”).

The advantage of decimal fraction is decimals all have the same common denominator in 10, so the arithmetic operations are easily carried out.

In a whole number you place a decimal point just to right of the units digit, followed, if you need it, by as many zeros as needed to show accuracy. The value of each place is one-tenth the value of first place to its left. And the place names to the right of the decimal point all end in ‘-ths’, e.g., 1.1 is one and one tenth (Also said "one point one"), 1.11 is one and eleven hundredths, etc.

Rounding-off Decimals: Particularly with decimals, rounding becomes necessary. Why? Decimals are fractions of 10; they are also the result of dividing denominator into numerator. Denominators that are not factors or products of 10 produce imperfect decimals that never end. Example, 1/3 = 0.333---. The --- indicates that the decimal continues forever; in this case, it continues as the 3, which can be signified by a bar over the digit that repeats but in many cases the digits repeat unpredictably and that is given by terminal 3 dots. These decimals need to be rounded to save time or for statistics as in rounding of whole numbers. For example, using the value for pi 3.14159265...., locate the digit in the round-off place. Here we decide on the ten thousandths, or 4^th decimal place, so locate by underlining: 3.14159265. If the first digit to your right of the round-off place is less than 5, the digit in the round-off place is unchanged (it is obviously not so in 3.14159265…, where the digit is 9); if it is 5 or more, the digit in the round-off place is increased by 1 (Here from 5 to 6) and the mixed-decimal, here the pi value, rounded off to 3.1416. (Most electronic calculators on the 4/5 sliding control do this automatically)

Examples: 3.249 to the nearest tenth is 3.2. In the case 473.28 to the nearest ten (not tenth) it becomes 470 (Note that when rounding to the left of a decimal point, the decimals are dropped). 2.4856 to nearest hundredths: 2.49. Next, 82,674.153 to the nearest hundred thousands: 82,700,000. And next, 64.982 to the nearest tenth: 65.0, and to the nearest unit: 65 (Note that 65.0 and 65 are the same value but in 65.0, the request was to round to the nearest tenth while in 65, to nearest unit). The 65.0 implies a higher level of exactness. And 3.99964 to the nearest thousandth is 4.000 (Note that because we were going from 3.999. to the next highest thousandth, all digits to the left of the thousandth decimal digit increase by 1 because the next highest thousandth after 3.999. is 4.000... .)

In rounding off mixed numbers in decimal form, if zero(s) replace(s) digit(s) to the right of the round-off place (e.g., 3.14159265 = 3.14160000), the terminal zeros imply a higher level of accuracy. If clarity is important, best to delete terminal decimal zeros unless the number is a measurement and you are implying statistical exactness. For example, in the case of pi, 3.1416 would be preferred to 3.14160000. That is because 3.1416 is already the result of rounding off and to add one or more zeros could mislead one into thinking the calculation accurate to 8 decimal figures.

Do not accumulate the rounding offs. For example, to round off 1.7149 first to the nearest hundredths and then to the nearest tens, round 1.7149 to 1.715, but then do not follow the usual rule, do not round to 1.72. The important digit is the 4 in 1.7149. It is 4 and therefore less than 5, so the round off digit 1 is not increased and the round off to nearest hundredth is 1.71 and not 1.72 as would have occurred if you started working with 1.715... .

Rounding Decimals in Computations: Addition (subtraction) sums (or differences) of approximate numbers contain no more accuracy than the least decimal place accuracy of the given approximate numbers. So, 3.14 + 15.812 + 0.5933 = 19.5453, sum should be rounded to 19.55 to accord with the two-decimal place 3.14.

In multiplication, the products of two approximate numbers (and quotients if division) contain no more significant digits than the numbers having the fewest significant digits.

Computation with Decimals: With addition and subtraction, the only point to remind on is to line up your columns with the decimal point as common reference. Also when a column of decimals adds up to 1.000… or more, the whole number digit 1 is placed to the left of the decimal point. For this reason, it is always a good idea to write decimals lower than 1.000... with a 0 to the left of the decimal point, e.g., instead of .678, write it as 0.678. Also when we write a mixed number as decimal, e.g., 1 1/2, or 1 5/10 = 1.5, it is understood that the value is 1 + 0.5; just as it is understood that 1 1/2 is the representation of 1 + 1/2.

The term ‘number of decimal places’ is as in the following examples: 0.25 has two decimal places; 0.054 has three decimal places; 14.5 has 1 decimal place; 0.5000 has four decimal places; and 167 has no decimal places. So ‘number of decimal places’ means number of digits or zeros to right of decimal point.

Dividing a Decimal by a Decimal: The divisor should be converted from a decimal to the smallest same whole number by moving the decimal point the appropriate number of places to the right, doing the same thing to the number it divides, e.g., Divide 2.368 by 0.32 is originally 2.368/0.32 and then move both decimal points two places to right for 236.8 divided by 32, and do the usual long division, respecting the decimal point of 236.8. Using a digital calculator, the correct answer shows as 7.4.

Multiplying and Dividing Decimals by Powers of Ten For each power of 10 multiplier, a decimal is moved one place to the right, e.g., 5.3 x 10¹ = 5.3 x 10 = 53; next, 5.3 x 10² = 5.3 x 100 = 530, and etc. And to divide a decimal by a power of ten is vice versa, e.g., 5.3 ÷ 10 = 0.53, next, 5.3 ÷ 100 = 0.053, and etc.

Changing fraction to Decimal is the simple division a/b = a ÷ b (e.g., 5/8 = 5 ÷ 8 = 0.625).

Changing a decimal to a Fraction, e.g., 0.4 = 4/10, and reduce to lowest term by dividing both numbers by 2 to get 2/5. Changing whole numbers and decimals to mixed number as fraction does not come up much, but here is an example: 7.04 is read "7 and four one-hundredths" (or "seven point oh four"), and write it as the mixed number 7 4/100 and reduce the fraction to lowest term by dividing both top and bottom by 4 to get 7 1/25, read as “seven and one twenty-fifth”.

Operations with Decimals & Fractions: Change to complete decimals or complete fractions. Example: 3.2 + 3/4 change completely to decimal for 3.2 + 0.75 and then add in usual way for 3.95 answer.

Complex Decimals are decimals combined at right end with fraction, e.g., 0.33 1/3. Since 1/3 = 0.333…, and 0.33 already has two decimal places, 0.33 1/3 is 0.33 plus 0.00333…, and you easily add to get 0.33333… . With 0.67 1/2, the fraction gives a clean 0.50 so the decimal equivalent is 0.675. (Continuing zeros optional since they don’t affect the value of 0.675)

Percent (%) is decimal with fraction expressed in hundredths. It replaces “hundredths” in the writing and saying, e.g., 0.34 is 34/100 or 34%; expressed “zero point 34” or “34 hundredths” or “34 percent.” (“Percent” literally means “per hundred”) Even when the decimal is not written as hundredths, you automatically convert it in making a percent, e.g., 0.5 to 0.50 to 50/100 or 50%; 0.34567 to 34.567% (Actually, a percent should always be rounded to either the nearest whole number or at the most, the tenths level because it is not used in calculations and not so exact; so 0.34567 ≈ 34.6% or 35%)

Percents greater than 100 are whole or mixed numbers, e.g. 500% is 500/100, or 5/1, or 5 x change; 112% is 1.12 and also as mixed number fraction 125% is 100% + 25%, or 100/100 + 25/100, or 1 +25/100, 1 + ¼, expressed as mixed number 1¼x change

Note that percent can mix with decimal or fraction notations, e.g., the NYC sales tax was 8.5% or 8 1/2 %. The 8 1/2 % or 8.5% becomes 0.085 as a complete decimal. This is a practical question because every day you may be called upon to convert percent to decimal, especially in figuring the actual cost of a VAT (value added tax). Percentages are only for visualizing fractions, decimals and ratios as parts in 100; they cannot be used directly in calculations, having always to be converted to equivalent decimal. For example, you will buy a book for $9.50 and the VAT is 8.5%. You calculate the added tax by $9.50 x 0.085 = $0.8075 ≈ +$0.81 or +81 cents. Get into the habit of mentally converting percent % to decimal. And commit to memory the percents of the simple fractions and vice versa so that you give them as 1/2=50%, 1/3≈33.3%, 1/4=25%, 1/5=20%, 1/6≈16.7%, 1/7≈14.3%, 1/8=12.½%, 1/9≈11.1%, 1/10=10%, 1/11≈9.1%, 1/12=8½%, 1/13≈7.7%, 1/14≈7.1%, 1/15≈6.7%, 1/16=6¼%, 1/17≈5.9%, 1/18≈5.6%, 1/19≈5.3%, 1/20=5%.

Percents expressed as tenths decimal are the same as per thousand. So 0.8% is 8 per thousand, and if you say 0.4% of the population is Hare Krishna, you see 4 shaved heads in a thousand non-s. Similarly as you go down the scale .01% is 1 per ten thousand, .001% is 1 per hundred thousand, .0001% is 1 per million.

Number Line & Signed Numbers: Focus on the below picture. Imagine it starting at 0 (Off the picture to your left) and then to 1 and next you see the 2, 3, 4, 5, 6 and to continue as high as you have patience to count. It is called a number line and the single digits numbers are 1 to 9 and the natural numbers include, except zero, every number you could count, and the whole numbers include 0. Infinity is the never reached outlier to your right, the end of all counted numbers.

A whole number is the exact number and should be written as pure digits with no fraction or decimal; integer is another word for it. The part of the number line in the above figure we see here, extending to your right, contains positive numbers and you can either write each number with positive sign, e.g., +1, or no sign at all. So when you see a bare number it has an invisible + before it. A negative number should be signed, e.g., the negative -2. An absolute number means the numerical value without sign. It cannot be written as an unsigned bare number. To indicate absolute, enclose in vertical bars [3].

Addition of two positive numbers, e.g., n + n, as commonly written is actually (+ n) + (+ n) = 2n. Adding a positive number does not change its sign, e.g., (+) + (+) gives + signed number.

Addition of a positive and a negative number, e.g., n-n as commonly written, or “subtraction,” is actually an addition of a positive to a negative, e.g., (+n)+(-n)=0.

With multiplication and division, minus x minus = plus (+), thus (–1) x (–2 )= (+2), while plus x minus or minus x plus = minus; thus (–2) x (+2 )= -4, and vice versa. Similarly in division (-1)/(-2)= 1/2, but (–1)/(+2) = -(1/2)

Concerning zero, anything plus or minus zero remains itself, e.g., 2+ 0 = 2 and 3 – 0 = 3. Multiplying anything by zero gives zero, e.g., 2 x 0=0, and dividing any number into zero gives zero, e.g., 0/2=0. But zero cannot be used as a divider (denominator in fraction) because its quotient (result of the division) infinity, e.g., 8/0 is impossible.

Order of Operations, Addition and Subtraction: In a combined calculation, first do the expressions in parenthesis then in the order of powers & roots and multiplication & division in order from your left to right, and lastly addition & subtraction in order from left to right. Concerning additions and subtractions, note that an expression such as 8 – 6 – 4 + 7 even when it stands alone must always be operated on from left to right. In subtractions, one must never switch the order of the numbers. Thus, 4+7 or 7+4 are the same, but 8- 6 and 6- 8 are not the same.

Grouping Symbols: ( ) Parentheses, [ ] Brackets, { } and Braces. Usually parentheses are preferred, reserving the other groupings for an operation within an operation, e.g., ( [8 + 4] – [9 – 7] ) ÷ 2 = (12 – 2) ÷ 2 = 10÷2 = 5.

EXPONENTS, Includes Logarithms
Exponents can be squares, cubes, powers: "square" is used when a number is multiplied by itself, "cube," is used when the number is multiplied by itself twice, (3 multiplications) and “power” may be used when any number is multiplied multiple times by itself. Thus 2 x 2=4 is expressed as 2²=4 and one says “Two square" or "two to the 2^nd power equals four.” The superscript number refers to the number of times a number is multiplied so 2³= 2 x 2 x 2 = 8 and so on. A general formula for every number is xⁿ=y where x is the base number, n is its exponent, or power, and the equation states that the base number multiplied by itself n times equals y. The base number n may be a fraction in which case the denominator of the n fraction is called the root of the base number. The x^1/2 is the square root or 2nd root of the base number x, and x^1/3 is the cube root, or 3^rd root, and so on. The numerator of the fraction remains the power. So x^2/3 is the cube root, or 3^rd root of x².

A negative exponent like x^–n is the same value of 1/xⁿ.

So 2^–2=1/2², or 1/4.

It can be proven that any number to the 1^st power always equals itself, e.g., 2¹ = 2, 3¹ = 3. Any number to the 0 power equals 1, e.g., 2⁰ = 1, 3⁰ = 1.

Square Root of Number: Perfect square numbers are composites that are the product of an integer multiplied by itself, like 4 as (2)(2) or 9 as (3)(3) or 16 as (4)(4). Stop at these factors and you are looking at square roots. So Sq Rt 4 is 2; Sq Rt 9 is 3; Sq Rt 16 is 4 and so on.

What about numbers not perfect squares? The above definition still holds and can be expressed by x² = (x) (x) and x is the Sq Rt of x². The symbol √ containing the x stands for Sq Root and if you have a more expensive electronic calculator you will spot the square-root key by that symbol and it is the easiest way to quickly find a Sq Rt. You can also use the Microsoft Excel (=....) or Google (...= the number followed by the exponent sign (^) followed by the in-brackets (1/2) sign, e.g. "4^(1/2)=2" on Google or "=4^(1/2) and hit the Enter key" on Excel). When x is like 2, 3, 5, not a perfect square we get square roots that are mixed fraction numbers as decimals that go on forever (e.g., Sq Rt 2 = 1.4142135… and we usually round off, for example Sq Rt 2=1.414, Sq Rt 3=1.723, Sq Rt 5=2.236.

When you come to Sq Rt 6, you may use knowledge of multiplication factors to easily obtain its Sq Rt from the Sq Rt of factors 3 & 2, e.g., Sq Rt 6=(Sq Rt 3) multiplied by (Sq Rt 2)=(1.732) (1.414)=2.449. This can be a useful way to get a higher Sq Rt when you do not have access to a Sq Rt calculator because one learns the lower Sq Roots by memory. Thus, continuing up, the Sq Rt 8 is (Sq Rt 4)(Sq Rt 2)=(2)(1.414)=2.818, Sq Rt 10=(Sq Rt 5)(Sq Rt 2) and so on.
The ancient Greek philosopher-scientists found a direct way to find any square root. As follows:

According to the Pythagorean Theory.

Right triangle

In a right-angle (90-degree angle ACB above) triangle, the sum of the square multiplication products of the right-angle lengths equals the square product of the diagonal line (the oblique, or hypotenuse, line c above). Here was the way to find square roots of numbers by marking out large right angle triangles on the ground and stepping the length of their sides and varying the lengths then using the formula Diagonal² = Height² + Length² and solving the equation for D. It can be seen that by varying the size of the triangle and measuring the height and length of the right angle sides of the triangle, any square root can be obtained by simply pacing out the distances.

The Sq Rt has positive or negative sign. For example 4 could equal either (+2)(+2) or (-2)(-2) so the actual Sq Rt 4 should be most accurately expressed as + or – 2 (±2) but we usually use the bare number.

There is no real number to express the Sq Rt of a negatively signed number like the Sq Rt of -4 since the only way to factor it is (-2)(+2) which cannot fit the definition of Sq Rt since it is not the same number multiplied by itself. In higher math the concept of negative number's Sq Rt is called imaginary number.

Orders of Magnitude: Prefix for Powers of Ten important in math and science.

10⁰ = 1, unit

10¹ = 10, tens, its prefix is deca (da)

10² = 100, hundreds, prefix hecto (h)

10³ = 1,000, thousands, prefix kilo (K or k)

10⁴ = 10,000, ten thousands

10⁵ = 100,000, hundred thousands

10⁶ = 1,000,000, millions, prefix mega (M)

10⁷ = 10,000,000, ten millions

10⁸ = 100,000,000, hundred millions

10⁹ = 1,000,000,000, billions, prefix giga (G)

10¹² = 1,000,000,000,000, trillions, prefix, tera (T)

10¹⁵ = 1,000,000,000,000,000, quadrillions, peta (P)

10¹⁸ = 1,000,000,000,000,000,000, quintillions, exa (E).

Example: giga: gigabyte is 1,000,000,000, or 1-billion bytes

Decimal Orders of Magnitude as Negative Powers of Ten in Scientific Notation:

1.0 10⁰ unit U

0.1 10^-1 deci- d

0.01 10^-2 centi- c

0.001 10^-3 milli- m

0.000001 10^-6 micro- μ (Greek mu)

0.000000001 10^-9 nano- n

0.000000000001 10^-12 pico- p

0.000000000000001 10^-15 femto- f

0.000000000000000001 10^-18 atto- a

The decimal orders of magnitude express a number between 1 and down to 0. The digits are lined up so that decimal point is always in the same column. The unit-1 boundary is written with a single decimal zero to right (e.g., 1.0) to indicate it is a pure whole number. In the case of decimal unit boundary, a zero is in the units position to left of the decimal point (e.g., 0.1) to make clear it is a pure decimal, not a mixed number decimal. As you may see by counting the zeroes, the number of the negative exponent, or negative power of 10 tells how many places to left of unit boundary you must move the decimal point.

Scientific Notation (SN): In SN the number is converted to the first order of magnitude (units, between 1 and 9.99) multiplied by the 10 power. For example the number two-hundred, forty-five, 245, becomes in SN 2.45 x 10², the decimal number 0.0245 becomes 2.45 x 10^-2etc. Because SN involves the superscript, which is inconvenient to type, the E system may be used; 2.45 x 10²becomes 2.45E + 2, and 2.45 x 10^-2 becomes 2.45E – 2 (minus 2) and so on.

With SN, say you need to multiply 2,450,000 x 2,000,000? Instead of worrying about those zeroes you immediately visualize 2.45 x 2 = 4.90 and since each is, at a glance, a million magnitude number you double the magnitude exponent (6 doubled to 12) and have the answer mentally 2.90 x 10¹², or 2.90 E + 12. And in big division with SN you often can spot a same factor cancellation that you would not normally notice with huge numbers of digits.

Expressing Fraction in Exponent Form: The exponents of the base ten as we approach and reach the zero power are: 10² = 100, 10¹ = 10, and 10⁰ = 1. As the exponent goes from 2 to 1 to 0, the number its base ten represents goes from 100 to 10 to 1. So the set of numbers that starts just below 1.000…, i.e., the below-1 fraction like 0.999, represented by fractions or decimals, has negative exponent of the base 10.

If we take a reciprocal fraction, expressed as 1/x, recall that the zero exponent of any number equals 1; e.g., x⁰ = 1; and that the 1-power of any number equals that number, e.g., x¹ = x, then 1/x = x⁰/x¹=x^(0-1)=x^-1. Thus a negative exponent number represents a pure fraction or decimal.

Translating the above equality to all exponents of base 10, it proves that when we express a fraction in exponent form, the exponent is negatively signed.

Logarithm derives from the Greek logos, ‘proportion’ and arithmos, ‘number’, i.e., ‘proportional number’, shortened to log. The following equality generalizes the relationship of any 1-or-higher positive number to the exponent-x to any number-N. It is the exponent function that anyone can check with real numbers.

y^x = N

The symbol x is the log of any number N, using as the base the number y for the exponent x. Thus for 2² = 4, the log is 2, i.e., the log of the number 4, using base 2 is 2; and for 10³ = 1000, the log of the number 1000 using base 10 is 3. Since log is written as a number, it, like any other number, may be given a decimal point, so in the example 2² = 4, the log 2 or 2.0 is equally correct. Note that the logs we have expressed in the above examples, 2 and 3 are whole numbers like 1, 2, 3, without any fraction or decimal attached. This makes it clear that in reading log number, the part to the left of the decimal point is the whole number exponent.

Now if we write log as isolated number, we have no way of checking whether it is correct or not because we don't know its base. If you tell me the log of 16 is 2, then I can figure out its base is the number 4: but not for larger numbers. Recalling from the above equation that we represent exponent or log number here as x, the base for the exponent as y and the real number that the log represents as N, the form of a log number is expressed as

x = log_yN

So for 4² = 16, we write log₄ 16 = 2, which would be stated “log to the base 4 of 16 equals 2”.

Logarithms to the base 10 – Common Logs: For each different base, a different set of log numbers represents the real numbers. Because base 10 logs have a special superiority, logs generated from the base 10 are called common logs. Now, look at base 10 exponents and the numbers they represent: the 10 to the minus one power = 1/10 or 0.1, and the 10⁰=1, and the 10¹=10, and the 10²=100, and the 10³=1000, and so on. So in base 10, the log of 0.1 = -1, the log of 1 = 0, the log of 10 = 1, the log of 100 = 2, the log of 1000=3, and so on. Or, in progressions, the geometric progression of the real numbers 0.1 to 1 to 10 to 100 to 1000 has been reduced in log form to the simplest arithmetic progression: –1, 0, 1, 2, 3, …. This was applied in slide rules because the set of whole numbers –1, 0, 1, 2, 3, … can be expressed linearly on a slide rule. Logs made the slide rule possible. But, today, computers and electronic calculators have retired the slide rule.

Natural Logs: A natural logarithm is a set of logarithms to the irrational number base given the letter symbol e and circa 2.718281828459… . So e^x = the number of its natural logarithm. As with all exponents, when x=0, e^x = 1 and its natural log is 0.0000 and when x=1, e^x = e so the natural log of e is 1.0000. However, other natural log numbers are unique to the exponent of e that each derives from. The usefulness of natural logs is not easy to explain. Intuitively we humans consider our whole numbers to be the “natural” or pure numbers because they derive from the 10 count system based on our fingers or toes. But nature has its own whole numbers that to us appear as the irrational number e and its exponents, which are the set of natural logarithms. Hence, “natural.” This comes out in higher mathematics and higher physics formulas and applications, and is another piece of evidence, in addition to the Golden Mean, that Nature has a system that differs from that which derived from humans. It is a hint of a perhaps true reality.

“Exponential”, as when we say “logarithmic increase”, means a continually accelerating increase as you would see if you took the function y=10^X and charted y on the vertical and 10^x on the horizontal. The following chart, showing world population increase, exhibits a logarithmic increase, or exponential increase of the number of humans (y on the vertical) against year (x on the horizontal)

Special Numbers - Primes and Perfects

Prime Numbers: If you look at the natural numbers after 1, you see two types of special number sets: The 1st type is the prime numbers: not a multiplication product of other natural numbers, primes are irreducible, each number being solely the product of itself and one; they are for numbers what atoms are for matter – the basic building blocks. In the first 10 natural numbers, 2, 3, 5, and 7 are primes. The number 1 is not considered a prime because, being the first and smallest full number there are no numbers it could be the product of. Real numbers other than primes are composite numbers, products of smaller numbers. In the first 10 natural numbers, the composites are 4, 6, 8, 9, and 10.

Knowledge of primes is useful in division and multiplication; it allows shortcuts, whereby 2-or-more-digit multiplications or divisions, which normally have to be done by the long written-out method, can be simplified to 1-digit calculations, easy mentally.

For example, the multiplication 101 x 16 usually would have to be done with pencil and paper or on calculator. But if it is seen as the primes 101 x (2)(2)(2)(2), the brain much more easily and rapidly processes the multiplication to 1616. Or the division 288/12 reduced to primes as (2)(2)(2)(2)(2)(3)(3)/(2)(2)(3) and by canceling equal factors above and below the double slash at a glance we get (2)(2)(2)(3), or 2 x 2 x 2 x 3 and the answer 24.

It has been noticed that primes do not occur with regularity or predictability and also the set of natural numbers goes on forever so the number of primes is infinite. If you discover a formula that will predict a new prime, a Nobel Prize in math should be created for you. At present the only way to determine large prime numbers is to work them out by hand or electronic calculator. Divisibility rules help: All even numbers except the number 2 are composites. All numbers whose cross-sums are 3, 6 and 9 are composites. And the other rules can identify many composites. But no test tells the less-than-obvious primes other than failure to produce the number as a product of smaller numbers.

Somewhere within the mystery of primes is a mathematical law of Nature that may benefit the world. It is waiting to be discovered. (cf. The Golden Mean ratio in art and the natural logs of e) Will you be the one to discover it?

The smallest primes are easy to see but finding primes becomes problem as they get higher. Before calculators, came the sieve of Eratosthenes.

Your Chart for the Sieve of Eratosthenes

1 2 3 4 5 6

2 3 4 5 6 7

8 9 10 11 12 13

14 15 16 17 18 19

20 21 22 23 24 25

26 27 28 29 30 31

32 33 34 35 36 37

38 39 40 41 42 43

44 45 46 47 48 49

50 51 52 53 54 55

56 57 58 59 60 61

62 63 64 65 66 67

68 69 70 71 72 73

74 75 76 77 78 79

80 81 82 83 84 85

86 87 88 89 90 91

92 93 94 95 96 97

98 99 100

Look at the 6 rows of the numbers, from 2 to 100. Circle the smallest prime 2 and then cross out all the even numbers; then circle the next prime 3, and cross out all its multiples. Then, circle the next prime, 5, and so on and by 100 you will have circled the first 25 primes. Now, look at what you have done and you should make the interesting discovery that, excepting 2 and 3, all the primes are located immediately before or after a number in column 5 (to its left or right). Column 5 consists of numbers all divisible by 6 and 3. This discovery greatly helps mathematicians locate new prime numbers above 100. As of year 2006, the largest prime by computer was 2^32582657 – 1, a Mersene number (See below) and a 9808358-digit number. But wait! Flash!

ORLANDO, Florida, February 5, 2013 — On January 25th at 23:30:26 UTC, the largest known prime number, 257,885,161-1, was discovered on Great Internet Mersene Prime Search (GIMPS) volunteer Curtis Cooper's computer. The new prime number, 2 multiplied by itself 57,885,161 times, less one, has 17,425,170 digits. (Still so as of 2017)

Goldbach, in the 1700's, based on years of trial and error, was unable to find an even number that could not be seen as the sum of two primes. But he could never mathematically prove that observation for numbers above which he had tested, and this remains the case. Today this is called Goldbach’s conjecture because it seems to be true in all cases that have been tested but has not been proved that it is always true as we go above the highest prime we have yet to find. If you can prove or disprove this conjecture for all primes, mathematical immortality awaits you.

In looking for primes higher than 100, we can still use the Sieve but it takes too long. The Roman Catholic father Marin Mersene in the 1600s stumbled on a method of finding very large primes. He did not have the exactly correct numbers, but his work was improved on and today its discovery uses his name for the Mersene primes which are prime numbers that may be generated from substituting in the formula 2^p – 1 where p is one of a series of the prime numbers: 1, 2, 3, 5, 7, 13, 17, 19, 31, 61, 89, 107, 127, 521, 607, 1279, 2203, 2281, 3217, 4253, 4423, 9689, …, 32,582,657 as of year 2008. Thus, smaller primes grow larger ones, àla Mersene.
Perfect Numbers: in 6^th century BC, the Greek, Pythagoras discovered a perfect number, whose all possible multiplication factors, including the number 1 but not the number itself, sum up to equal itself. The smallest is 6 whose multiplication factors are 3x2x1. The next larger is 28 whose possible multiplication factors are 14x2x1 or 7x4x1, giving a sum of all the possible factors, 14+7+4+2+1=28.

How to check if a number is perfect? Halve the number until and including the first odd one then continue the series with the number 1 and doubling until the highest doubling that is below the odd halving and add all. If it is a perfect number, the sum should be the tested-for number, The next perfect is 496 (248+124+62+31+1+2+4+8+16=496). The ancient Greeks knew of four perfect numbers; the three already given and 8,128, and notice they are all even numbers and end in 6 or 8 in a regular alternation. This does not continue with higher numbers.

If you inspect the first 4 perfect numbers 6, 28, 496 and 8128 by breaking them into their multiplication factors, you see: 6 (2)(3), 28 (4)(7), 496 (16)(31), and 8128 (64)(127). The 5^th perfect number 33,550,336 was found about A.D. 800. By 1965, twenty perfect numbers had been found and then in 1969 and 70, with a computer, and using the discovery that the perfect numbers are all generated from the expression 2^p−1× (2^p − 1) where 2^p − 1 is a Mersene prime, a 17-year-old, Roy N. Ferguson found the next three perfect numbers. The highest, the 48^th perfect number, is 169,296,395,270,130,176, was generated by computer in 2013. Who’ll try for next high?

A rational number comes from a type of fraction and also includes all whole numbers. It is a number that can be written in the form of a/b, where, a and b are whole numbers and b does not equal 0, and the decimal formed from a rational number fraction must be either terminable or repetitive with the same terminal digit. Examples are the number 3 because it can be expressed as the fraction, 3/1 or terminating decimal 3.0; the 2½ because it can be expressed as 5/2 or terminating decimal 2.5; and the 1/3 because it can be expressed as the same digit-repeating decimal 0.333333---; and also square roots that are whole numbers, e.g., sq rt 25= 5=5/1.

An irrational number is a number that gives a non-terminating, non-repeating decimal. Examples: square roots of prime numbers or those that factor to give a square root of a prime number, e.g., sq rt 2 = 1.414213562… or sq rt 8 because it factors to sq rt 4 x sq rt 2 = 2 x 1.414… = 2.818…; the geometric constant π= 3.1415926535….

Together, the rational numbers and the irrational numbers form the set of real numbers so called because there is a one-to-one correspondence between real numbers and the points on a number line. In contrast, imaginary numbers (square roots of negative signed numbers) cannot be placed on a number line because there is no correspondence between, for example, the sq rt -4 (for which no actual number can be found) and a point on any imaginable number line.

STATISTICS

All of us thinking in a scientific way want to accurately give numbers to our observations. But the problem is: How typical are the numbers? That is the question for statistics. If we meet three 18 year-old boys who are German and all taller than 1.9 meters, are they typical or not? Is it accurate to say after the meeting: "Eighteen-year-old German males are taller than 1.9 meters?" Here is where central tendency comes in.

First is to get more than several sample measurements. In science the number of measurements needed is determined by statistical method. Also, whatever we measure ought to be specified. If studying human body height, we should specify separately male or female and age. The fewer the specifications, the less useful information we get (e.g., to get the combined measurements of all Germans, male and female regardless of age, becomes confusing because of man-woman differences). So we specify height of German males, ages 18-20. We then measure 10 randomly selected in that set. “Random” here would mean we select by having the names of all German boys between 18 and 20 placed in hat (A large hat!), shaking the hat well, and selecting by moving our hand around at random and withdrawing ten names. A “nonrandom” selection would be to visually select the boys in face-to-face meeting where we run a risk of selecting short or tall one and getting inaccurate data.

Here are height measurements in centimeters of the randomly selected ten German boys: 190, 170, 165, 180, 179, 181, 167, 156, 191, and 172. By looking at the 10 measurements you get a rough idea and seeing the range 156 to 191. The data may be summarized by 3 values that give central tendency: the mean, median, and mode.
The mean, or average. Add up the ten heights and divide by the number of measurements: 1751/10 = 175.1 the mean. So German boys in this category average near 175 cm height. But sometime a mean is affected by a few extreme measurements, here the 156, 191 and 190. If you dropped them, the remaining 7 would sum to 1214 giving average near 173.4. The mean, especially when its total measurements are few, can be badly affected by just one or two high or low outliers and give a less than accurate central tendency.

To correct for this we have the median, obtained by lining up the measurements in order of size and selecting the one or two at center. Here with 191, 190, 181, 180, 179, 172, 170, 167, 165, 156, the central measurements, or median range, are 179 and 172. If we had an odd series there would only be one median; in this even number there are two so we average them (179 + 172)/2 = 351/2=175.5, which gives us a median quite close to the mean of the 10 measurements and causes us to have more confidence in that mean as an accurate measure of typical heights.

Another measure is the mode, which is the number that comes up most frequently in a series of related numbers. Here there is no mode since no number repeats itself. The mode occasionally is useful in pointing attention to a sub population of measurements due to unusual cause. The range gives an idea of the degree of dispersion.
Statistically Significant What is it?

Standard Deviation: The above simple arithmetic measures of central tendency give idea of how typical one's data are, but for drawing scientific judgment on meaning of differences in experiments one needs the standard deviation. In a small population where we can measure every person, it is the population standard deviation. In most cases this is not possible or practical so we select a random sample and get a sample standard deviation, which is the usual SD in most experiments. The calculation for SD starts by taking a series of measurements, obtaining the mean, and then subtracting each individual measurement from the mean, squaring the difference, and adding the sum of the squares. Then for population SD we divide that sum by the number of measurements, and get the square root of that result. But for sample SD we divide the sum by the number of measurements minus 1 (n – 1). In the case of the measurements of height of a sample population the calculations go as follows:

Cm. height in left col; diff. from the mean in 2nd col. and square in 3rd

181	4	16
175	2	4
174	3	9
178	1	1
Ave.= 177

The sum of the squares of the differences of each measurement from the mean is 30. Since we measured a sample and not the whole population we divide the sum by number of measurements minus 1, or 3 and obtain square root of quotient on calculator and it gives the SD, or as follows

30/3, or 10, and the sq root of 10 is (+ or –) 3.2 rounded, the sample SD. So in this experiment mean height with SD is 177±3.2.
The SD is useful because testing with large populations has shown that the range of 1 SD (here further rounded and ranged to 174 to 180) includes 67% of all the tested population; the range of 2 SD (here 171 to 183) includes 95%, and the range of 3 SD (here 167 to 187), 99.7%. The reason for these very wide ranges is because we have a very small sample size. In a usual experiment it would be a bigger sample and it would give smaller SD ranges. In the simplest experiment, sample measurements should not be less than 10.

Scientists have made it a rule that, in a hypothesis, which depends on differences in measurements or observed numbers, the experimental value must differ by at least 2 SD from the control value for the difference to suggest possible causation also called “statistically significant.” Note that this still leaves the probability that, if one makes repeated experiments, similar differences at the 2 SD level could occur by chance alone 5% of the time. So it does not prove a hypothesis. But it makes the hypothesis likely enough that we start to take it very seriously as an explanation. If we can’t prove a result at the 5% level (differences less than 2 SD), we consider that our experiment has been indecisive and possibly not worth repeating. But if we get a difference at the 2 SD or more level, we feel we have, practically, proved the hypothesis.

An example to show the use of SD might be answering the question: Do 18 to 20-year-old Italian boys differ from same age German boys in height? Here there is no control; just a difference between mean and SD measurements of two groups.
An experiment where we use a control would be to answer the question: Do feeding 15-year-old German boys vitamin E supplements have any effect on increasing their height? Here, the control group would be the total population (very strictly a group similar in all ways except vitamin E) of German boys, ages 15, and the experimental group would be the set of German boys to whom we fed a set dose of vitamin E from age 15 and then measured the heights of both groups at age 20. I do not work through the numbers here; Why don't you do it from your imagination?

Geometry

First about triangles, the Pythagorean Theory. We have above mentioned this, in passing, under square roots.

Right triangle

In a right-angle (90-degree angle ACB above) triangle, the sum of the square multiplication products of the right-angle lengths equals the square product of the diagonal line (the oblique, or hypotenuse, line c above). As mentioned, it was a way of finding square roots of numbers by marking out large right angle triangles on the ground and stepping the length of their sides and varying the lengths then using the formula Diagonal² = Height² + Length² and solving the equation for D. It can be seen that by varying the size of the triangle and measuring the height and length of the right angle sides of the triangle, any square root can be obtained by simply pacing out the distances.

Note the 3-4-5 is ‘magic’ number sequence for a right-angle triangle because 3² + 4² = 5² so a right-angle triangle whose two right angle sides are 3 and 4 inches, will predict the hypotenuse side to be 5 inches. It is one of Pythagoras's magic triangles. (The sides of the right triangles can be other whole numbers) Some other points about triangles: The sum of the degrees of 3 angles of any triangle is 180⁰ so if you know the degrees of 2 of the 3 angles, you can find the 3^rd by subtracting their sum from 180. A right angle is 90⁰, a.k.a. perpendicular (orthogonal) angle. That is important knowledge because you can check it by a glance. A triangle with two equal length limbs (isosceles triangle) has equal angles at the base and so the angle of the apex (top) is 180 minus 2 x the angle degree of one base angle.

Areas: Triangle = One half height x base; Rectangle = height x length; Square (rectangle with all sides equal) = (length of side)².

The Circle

Search Results

Featured snippet from the web

radius diameter circumference: circle/diameter = pi = 3.14159...

About Circles: If the line of the radius (r) is extended to the opposite point on the circle from where it started, you have the diameter (D) of the circle. D=2r (r is radius, or half diameter of the circle). The circumference (C) of a circle is the measured length of the circle’s outline. (Visualize the circle line as a flexible wire and if you cut it at 1 point and stretch it straight and measure its length, you have its C) Note the formula for circumference C = π·D and that π (Pi) is a constant value that has been obtained by dividing circumference of any circle by its diameter (π = C/d, or C/2r; another value that the ancients could pace out). Pi is an irrational number, meaning its series of decimal digits never end. The area of circle is π multiplied by r².

Volume (3-Dimensional) and Area (2-D) are important geometric concepts for a scientific education. Above, you saw formulas for some common areas. Note they are products of 2 dimensions of physical reality: width and length. The 3^rd dimension is height (off the screen or paper, above and below it). The height, length, and breadth, or width, are relative terms for physical units of measuring lines in one of the three different 90-degree directions, or dimensions. The important concept is that each is defined as being at right angles in space (orthogonal, 90⁰ angle) with reference to the other.
Think of the two dimensions of width and length in the plane of this screen or of a paper you draw on. Then imagine a third line that intersects where the 2 lines intersect but comes directly out of the screen right at you, in your face, and also goes directly behind the screen in back, and you have the 3^rd dimension of our physical world. Each 2-dimension geometric flatland figure when enlarged into the 3^rd dimension, becomes a solid figure, e.g., the square becomes a cube, the circle becomes a sphere; and the area of the square, obtained by multiplying the length and width of its sides (side² multiplied by its extension into the 3rd dimension), when multiplied by the height of the cube, becomes the volume of the cube, (side)³ because we multiply the 3rd side as the line that we imagine coming out of our screen by the area. For the circle, we imagine our 2-D circle standing upright and revolving through 360⁰ (Or blowing it up with air like a balloon) to give a sphere.

Visualizing Size of Sphere from Cubic Measurement: A sphere as most of us know is a circle carried into our 3rd dimension. With sphere measurement, imagine what its actual size is. For example, recently I read that the largest flight balloon ever built had a volume of 12-million cubic feet. It sounds a huge measurement but what actually would such a balloon appear when measured in feet across? Looking at the volume equation for sphere, we see V =4/3 πr³. Since π=3.1416, Volume = 4.1888r³, and solving for r we get r³= V/4.188. Substituting 12,000,000 ft³ for V and dividing, we get r = the 3^rd, or cube root of 2.8648 x 10⁶ feet, which by Google or Microsoft Excel formula, computes to 142 feet, so the diameter of the balloon would be 284 feet across. Thus, you see, we have translated the unimaginable but gigantic-sounding 12-million cubic foot balloon to the easily visualized 284-foot diameter balloon. (For those like Americans who do not use the metric system) The general solution is simply to divide whatever cubic feet a balloon is given in, by 4.1888 and, using a computer Google or Excel, get the cube root of the quotient to give diameter of the balloon in feet and, if needed, convert to meters.

The Fourth Dimension: in a 3-D world, no reality contains more than 3 dimensions; we cannot imagine 4 dimensions.

Get typing paper, pencil, ruler, and scissor. Be sure the pencil is sharpened to fine point. Make a dot in mid paper. That is a point. It may have microscopic thickness but we can consider its dimension zero. Make a 2nd dot, a few inches or centimeters from the 1st dot. Connect the 2 dots by pencil line with ruler. Now you have a line, or segment. It has one dimension – length. Now, in mind's eye, visualize that line sweeping up or down (or sideways if you drew the line vertically on the paper) in a direction perpendicular (exactly 90 degrees) to itself but in the plane of the paper. If you visualize the line sweeping perpendicular for its own length (same length as the line itself), you see a square. So now draw it. Note the square has 4 points at corners and 4 lines on sides. It is flatland, exactly in the plane of the paper, 2 dimensions. The 2 dimensions are length and width but no height, and the square or rectangle or polygon (many sided enclosed 2-dimension figure) or circle are its figures.

OK. Now get ready to move into the 3^rd dimension. Look at your square and think of it as an inflatable right-angled building that rises up out of the paper in the exact 2-dimensional shape of the square. But now with its rising, it occupies the 3^rd dimension – height. If it rises up the exact height of its length and width, you will see a cube coming out of paper. (The cube could go below paper too) The cube has 8 points, 12 lines (or sides), and 6 squares enclosing its inside content. The numbers of points, lines, and squares as we increase dimensions show an interesting relation to the dimension number. For 0, 1, 2, and 3 dimensions, there are 1, 2, 4, 8 points; 0, 1, 4, 12 lines, or segments; and 0, 0, 1, 6 squares. For points the progression obviously is simple doubling, which may also be expressed as 2ⁿ (read “two to the nth power, and n= dimension number, e.g., 2⁰=1, 2¹=2, etc.)

The progression of the number of lines with increasing dimension is seen from the points. The zero dimension 1 point x (multiplied by) 1 (the 2nd point) gives the 1 segment or line of 1st dimension; the 1st dimension 2 points x 2 gives the 4 segments of 2nd dimension; and the 2nd dimension 4 points times 3 gives the 12 segments of 3 dimensions. The formula for this progression is p_(n-1) x n = s_n (where n is the dimension you are calculating segment number for, p is the number of points in the subscript dimension, and s the number of segments in the subscript dimension).

The relationship of number of squares to dimension is less obvious but even the two dimensions that have squares show that by doubling the number of squares of the 2^nd dimension (1 x 2 = 2) and adding the number of segments in the 2^nd dimension (4) one can predict that the equivalent 3^rd dimension solid cube will have 6 squares.

So far so good! Now we come to the 4^th dimension and we run into trouble with our mind's eye! We can visualize a figure equivalent to zero dimension dot, a 1^st dimension line, a 2^nd dimension square or a 3^rd dimension cube; but, when we try to imagine seeing a cube moved through the 4^th dimension to form a hypercube, or tesseract, the word for such an unimaginable object, we fail because our 3-Dimension reality contains no further dimension to see ourselves move our figure into.

We can calculate the numbers of points, lines, squares and cubes of the tesseract based on the arithmetic formulas we derived from point, line, square and cube for the first 3 dimensions. Thus, for number of points of a tesseract using the 4 of 4^th dimension, we get 2⁴, or 16, for number of segments or lines, we multiply the points of a cube (8) by 4 for 32; for number of squares, we double the number of squares in cube (6x2), add number of segments of a cube (+12) and get 24 squares in a tesseract. As for how many cubes a tesseract should contain, we have no formula for that but mathematicians have analogized, the formation of a tesseract, considering its formation by moving its 8 corners through a 4^th dimension to make 8 cubes in a tesseract, just as we produce a cube by moving a square through the 3^rd dimension by its 4 segments or lines to make 6 squares as walls of the cube. So we have the dimensions of a 4 dimension structure that remains unimaginable and that is as far as we can go.

The time measurement has been proposed as the 4th dimension. Perhaps if you took the above cube and allowed 100 years to pass, and controlled for the various movements in space that might affect the cube, its video representation photographed every instant might give an idea of what a tesseract looks like. As for me I cannot imagine time or anything else as a dimension, but then I am a 3-D human so the 4-D world is unimaginable.

In 2018, a movie came out titled A Wrinkle in Time from the popular 1960s science-fantasy novel of the same name by Madeleine L'Engle, in which the central part of the plot involves what is called tesseract but it is stated to be in the 5th dimension. This ought to ruin the story and the movie for those of us who are purist for fact. Tesseract is a 4th dimensional cube. But for a much better novel built around a 4th dimensional tesseract, I recommend The House of Many Worlds by Sam Merwin Jr, first published in 1951 got on Amazon.com.

Visualizing Acres: Getting back to reality, the measurement of area is in square unit, e.g., square inch or square centimeter. Since to get an area we are multiplying a length by a width and both are in the same unit of line measure (line x line) we get a square, (line)² of that measure whether it be inch or centimeter. An old fashioned measure is acre; it is still used much in English for size of land a house is built on. But, unless one is a landowner, those who talk or read about acre really cannot visualize the size referred to. Here is an easy method. First, its basis!

The acre is equal to 43,560 square feet. OK, now go to Google or Microsoft Excel to find the square root of 43,560, which is the square footage of the acre, and hit your Enter key and you should see 208.71032 (feet). So next time you hear or see “acre”, you may picture a square plot of land c.209 feet on each side. In the real world, plots of land are not perfect squares; still, it is better than before when you had no idea what an acre looked like. What about plots of acres? For any plot of acres, divide its acre number by 640, key in square root for it, and your screen should show the mileage of 1 side of a square of the said acres. And if the mileage is too small to visualize, you can always multiply its decimal fraction by 5,280 feet and get it to feet.
By the way, the historical basis of acre was the amount of land that could be plowed in 1 day and it was a rectangle space, one side of which was 10-multiplied by the other side, eg, 22 by 220 yards. But that is just a historical note today.

I want to mention Rhombus here because in Notebooks 9 you will run into rhombic and the prefix rhombo-, which are geometric-shape descriptives in neuroanatomy. A rhombus is a simple quadrilateral (4-sided) polygon (box-shape) whose 4 sides all have the same length and that differs from a square in that the sides are angulated and parallel; in other words, diamond-shape.

End of Numbers Section. To read on now, click 2.6f Temperature Calculations - Celsius/Fahrenhei...

Physician's Notebooks

Pages

Thursday, September 23, 2010

2.6e Numbers for Healthy Lo ngevity

Search Results

Featured snippet from the web

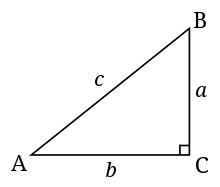
No comments: